Progress on property dispute in North Kaneshie

The property dispute reported on in November is surging towards conclusion.

The segment of the external wall which formerly went through the house, exiting through the crude-looking doorway, has been removed (except for one small section inside the house not visible from this angle).

Now if only they could do something about the road.

A framework for applying computation theory to the study of earth history

Introduction

Today we look at a different means of characterizing the complexity of geological data sets using Crutchfield's (1994) "epsilon-machine" concept.

Crutchfield (1994) presented a framework for attacking the following questions. How do we discover anything new when everything we can describe must be expressed in the language of our current understanding? How does innovative behaviour arise from deterministic dynamic systems?

The kernel of Crutchfield's (1994) paper is that the "work" of complex natural systems can be likened to computation, and is capable of being studied in the same manner. Complex systems may thus be understood in terms of how they process information. Computational theory, then, lies at the very centre of the study of complex natural systems.

Computation has an heirarchical architecture, and it is this structure that makes complex behaviour possible. The necessity of heirarchies in the generating innovative or complex behaviour has been previously noted by Hofstadter (1979) and Casti (1992).

The epsilon machine approach is one in which we organize our observations of natural systems in a heirarchical architecture in order to enhance our understanding of them. Today we will look at some examples of this approach using geological time series.

Most of the images below are from the presentation I made last year at GAC (pdf).


First of all, let us consider how an earth system might be considered to be carrying out computation. Consider climate.



The global climate system can be viewed as a machine that operates on inputs, which include the amount of radiation received from the Sun; its latitudinal variations (which change with key astronomical parameters); and possibly other inputs beyond the experience of this author. We don't necessarily know all possible inputs.

The machine uses an unknown series of subroutines, each of which are influenced by factors such as the strength of various ocean currents, the pattern of overall atmospheric and oceanic circulation, the configuration of the continents, levels of atmospheric dust, atmospheric composition, etc. To make matters more interesting, some of these parameters are themselves outputs of the system.

The outputs of the system include the obvious ones like global ice volume, and global temperature distributions. Realistically, however, they also include a myriad of local temperatures, ice conditions, water temperatures, wave conditions and so forth. Like the inputs, there are more outputs than can be conveniently itemized. From the standpoint of understanding the workings of the computer, our opinions on important global parameters (say, ice volume) may not actually be significant.

To make things more challenging, we only perceive the present outputs of the system directly. We have to infer previous outputs from the geological record, which does not necessarily record the outputs cleanly. Normally we infer the outputs through the use of paleoclimate proxy data (frequently isotopic ratios, but sometimes also ratios of certain organic molecules, or the chirality of certain organic isomers. Implicit in this approach is the assumption that the relationship between the output and its proxies are constant, which is by no means certain as most of these proxies are related to the energy relationship between various organisms and their immediate surroundings: a relationship which may be expected to vary through time as as innovative behaviours are selected for by competitive or environmental pressures.

Even after the geological information is recorded (usually in sediments), it is subject to alteration by tectonic activity, or changes in temperature, or chemical alteration, and it can be challenging to recognize and correct for such changes.

In effect the geologist is trying to work out the computational details of an unknown program running on an unknown operating system with an unknown computational architecture. Some of the inputs are known, but an unknown number of additional inputs also exist, some of which may be more important than the known inputs. Some outputs are known, but these may have been altered to an extent which can be difficult to ascertain.

Is there any way we can decide which of the many possible outputs of the system to study?

The answer is surprising. According to Packard et al. (1980), it doesn't matter. All outputs of the system contain all of its information, albeit not necessarily in a form that is easy to recognize. As specialists, it is to our benefit to concentrate on our favourite record (or records). So I will show you how to construct epsilon machines using two of my favourite time series, and you can follow along with your own.

Epsilon machine construction

The process for constructing an epsilon machine is as follows:

1) define predictive and successor states - these are repetitive strings within the data, which are followed in a more or less predictive fashion by other repeated strings.

In the figure above, I have identified two repeated sequences, labelled A (1,2,3,2), and B (2,3,0,1). In the series above, assuming that the sequence of data runs left to right, it appears that A is the predictive state and B, which follows it, is a successor state. Since we are only looking at a fragment of the total data stream, it is just as likely that B is the predictive state and A is the successor state.

2) Construct the epsilon machine by calculating and recording the probabilities of all observed relationships between predictive and successor states.

3) If there are more than one epsilon machine of a given order, then each may itself be a predictive (or successor) state of the next higher order. These higher-order predictive/successor states can be used to define a higher-order epsilon machine in the same manner as in step 2.

In the diagram above, we have defined three epsilon machines from our hypothetical data set. Their existence allows us to construct a second-order epsilon machine from the three first-order epsilon machines.

This third step is where the heirarchical nature of epsilon machines can be realized. Note also that as in mathematical induction we are not specifying the order, so this step is repeated, and epsilon machines of successively higher order are defined until the data gives out. Given sufficient data, you may construct epsilon machines of arbitrary order.

Predictive states in paleoclimate proxies

Let's look at our data.

Here are two well-known data sets: the magnetic susceptibility record from a long loess record, which is a proxy for the strength of the Himalayan monsoon (Kukla et al., 1990); and the deep-sea d18O record, which is a proxy for global ice volume (Shackleton et al., 1990). The d18O record has been available from NGDC servers--the magnetic susceptibility record was obtained from the original publication, and digitized by this author.

The first obstacle in applying this method to geological time series is in defining predictive/successor states. The uncertainties in typical geologic records are so great that strings of identical observations are extremely unlikely. We need to find another approach to defining predictive and successor states.

Herein I propose that we determine predictive and successor states from probability density plots of two dimensional reconstructed phase space portraits. The particular approach we will use involves the use of the time delay space (Packard et al., 1980; Mañé, 1981). 

Sequential viewing of the probability density plots for gives you an animation effect. The key here is to note that the regions of high probability are relatively constant throughout the Quaternary and are interpreted to represent areas of Lyapunov stability. These regions of stability shall be our predictive/successor states.

Epsilon machines from the paleomonsoon proxy

In the early Quaternary, we observe three predictive states in the probability density plot of the two-dimensional reconstructed phase space portraits of the paleomonsoon proxy. These have been labelled M1, M2, and M3.

 Depending on your browser you may need to click on the above figure to see the magic.

Nice simple epsilon machine (μ1) for the paleomonsoon proxy in the early Quaternary. Som of the simplicity has arisen from a long interval in which M2 appears to be the only stable state, although close inspection of the record actually suggests a limit cycle around M2.

Three more predictive states are apparent in the Late Quaternary, leading to a more complex epsilon machine we will call μ2. The sequence of observed first-order epsilon machines is μ1, μ2, which is not a particularly inspiring second-order epsilon machine, but perhaps the Quaternary isn't finished yet.

Epsilon machines from the ice volume proxy

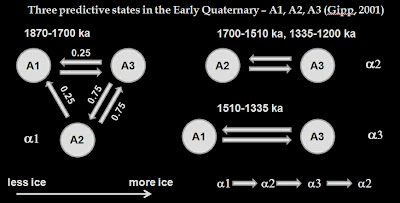
In the early Quaternary, there are only three predictive states--labelled A1, A2, and A3 (Gipp, 2001). For the purposes of this article we are ignoring the limit cycles seen here. A fourth predictive state (A4) appears in the mid Quaternary, and the fifth (and sixth) appear only in the Late Quaternary. Also in the Late Quaternary, predictive state A1 all but disappears.

We observe three distinct interrelationships (epsilon machines) between our three predictive/successor states active during the Early Quaternary. The epsilon machines have been labelled α1, α2, α3 and each are active during different portions of the record (it may be that α2 and α3 simply represent limit cycles).

What does this tell us? From a computational standpoint, for α1--if the first predictive state was A1, then the next successor state is always A3. From A3, there is a 0.25 probability that A1 is the next successor, otherwise it is A2. From A2, there is a 0.75 probability that A3 is the next successor, otherwise it is A1. Given this information, one can use a stochastic process to generate a string of successor states that satisfy the criteria of the epsilon machine, but which would not necessarily be the exact sequence of observed states. 

A climate model of this stage of the Quaternary could be readily tested against the observed model by testing to see whether there is a recognizable string of predictive/successor states in the output, and whether they satisfy (approximately) the observed probabilities (we should recognize that our observed probabilities may be relatively poor estimates of the actual probabilities). There are statistical tools for demonstrating the likelihood that the probabilities generated by the computer model are in agreement with the observed probabilities.

During the mid Quaternary, ice volume state A4 appears for the first time, and the epsilon machine (α4) shows increased complexity.

In the late Quaternary, the appearance of predictive state A5 ushers in a new, more complex epsilon machine I have called α5. The asymmetric sawtooth nature of glaciations in late Quaternary are reflected by the high probability of a rapid drop from A5 to A2 (p = 0.57), A3 (p = 0.14), or A1 via A6 (p = 0.14).

The five first-order epsilon machines we define through the Quaternary may be used as predictive/successor states and used to construct a second-order epsilon machine. There are not enough transitions to establish probabilities for transition from one second-order state to another, so our knowledge of this machine is lacking. At present it appears to be: α1, α2, α3, α2, α4, α5. It is a little more interesting than the second-order epsilon machine for the paleomonsoon proxy, but not completely satisfying. Nevertheless, it is still useful as a descriptor for the climate system, and can certainly be used to test computer models of climate.

Examples of testing models against the geologic record

Computer models of ice volume may be tested by determining the predictive or successor states from the model output, and comparing the resultant epsilon machines to the observed system. For a computer model to be considered successful, it should generate more than one first-order epsilon machine.

Let's try it.

Model 1: Parennin and Paillard (2003) model for Late Quaternary glacial cycles recognizes three metastable states: 1) an interglacial regime; 2) a mild glacial regime; and 3) a full glacial regime. The steps from one regime to another are driven by variable responses of the ice volume to northern hemisphere insolation modified by the extant ice volume. When in the mild glacial regime, ice volume does not drop suddenly in response to increasing insolation; nor does the system evolve back to the interglacial regime. Each transition from one state to another represents an irreversible change. Deglaciation only occurs from the full glacial regime in response to an increase in insolation.

The epsilon machine for this model output is summarized below.

. . . where I represents the interglacial regime, M represents mild glacial regime, and F represents the full glacial regime. In terms of the predictive/successor states used in the epsilon machine method, I corresponds to A2, M to A3, and F to A5.

The epsilon machine from the Paillard (2001) is simple, but no innovative behaviour is likely due to its tight definition. As there is only one first-order epsilon machine, there will clearly be no higher-order epsilon machines. Nor is it clear how to extend the model backward to the mid or early Quaternary.

A model constructed by Saltzman and Verbisky (1994) uses multiple feedbacks ('+' means positive feedback; '-' means negative) in order to generate a results which could be tested against a three-dimensional phase space defined by global ice volume, ocean temperature, and amospheric carbon dioxide. Interestingly, the resultant model successfully captured the multistability that characterizes the data; albeit one in which only two predictive/successor states are observed. The model was only run over a limited portion of the Late Quaternary, during an interval in which only states A2, A4, and A5 are observed.

If Kauffman's (1993) assertion that the number of metastable states (or Lyapunov-stable areas) in a complex system is a function of the square root of the number of parameters holds true, then the Saltzman-Verbitsky model has between about 1/2 and 1/9 of the number of parameters necessary to generate a model as complex in behaviour as the observed Late Quaternary ice volume proxy.

Nevertheless, the generation of multistability in this model represents a form of innovation (such multistability is not explicitly defined in the model), which places this model in my view above the Parennin and Paillard (2003) model--and indeed above virtually all other models of Late Quaternary climate encountered by this author.

Improperly nested epsilon machines in the geologic system

The observed first-order epsilon machines above (alpha-1 through alpha-5) can themselves be considered predictive or sucessor states (we shall call them second-order states to distinguish them from A1 through A5). The sequence of second-order states defines an epsilon machine of the second order. The second order epsilon machine, once completed, represents a third-order predictive or successor state, and given the number of such states, it may prove possible to define a third order epsilon machine.

Successively higher-ordered epsilon machines can be defined as long as there is data to support them. In theory there may be no limit to the order of machine that may be identified in geologic records; but in practice data are limited and we do not expect to easily rise above the second order epsilon machine.

For climate change, higher-order epsilon machines are expected to be related to higher-order cycles within earth history. Higher-order epsilon machines may relate to major tectonic events, and it may eventually prove possible to define epsilon machines of order W, related to Wilson cycles. Beyond that, would be a larger epsilon machine related to the formation (and eventual destruction) of Earth.

The heirarchy of computational processes necessarily reflects the heirarchy of geological processes. But these heirarchies are not perfectly nested. As related in the introduction, some of the results of Wilson-cycle dynamics feeds into the atmospheric circulation. As a consequence, the heirarchies are not nested, they are tangled. Such tangling of heirarchies is a requirement of innovative, complex behaviour (Hofstadter, 1979), and is no doubt the reason that such behaviour is a feature of geologic systems. 

References

Casti, J., 1992. Reality Rules: Understanding the world through mathematics.
Crutchfield, J. P., 1994. The calculi of emergence: Computation, dynamics, and induction. Physica D 75: 11-54.
Gipp, M. R., 2001. Interpretation of climate dynamics from phase space portraits: Is the climate system strange or just different? Paleoceanography, 16, 335-351.
Gipp, M. R., in press. Imaging system change in reconstructed phase space: Evidence for multiple bifurcations in Quaternary paleoclimate. Paleoceanography.
Hofstadter, D., 1979. Godel, Escher, Bach: An Eternal Golden Braid.
Kauffman, S. (1993), The Origins of Order: Self-Organization and Selection in Evolution, Oxford Univ. Press, New York, 734 p.
Kukla, G., Z. S. An, J. L. Melice, J. Gavin, and J. L. Xiao, 1990. Magnetic susceptibility record of Chinese loess. Trans. R. Soc. Edinburgh Earth Sci., 81: 263-288.
Mañé, R.,1981. On the dimension of the compact invariant sets of certain non-linear maps, in Dynamical Systems and Turbulence, Warwick 1980, edited by D. Rand and L. S. Young, pp. 230-241, Springer-Verlag, New York.
Packard, N. H., J. P. Crutchfield, J. D. Farmer, and R. S. Shaw, 1980. Geometry from a time series, Phys. Rev. Lett., 45, 712-716.
Paillard, D., 2001. Glacial cycles: Toward a new paradigm. Reviews of Geophysics, 3: 325-346.
Parrenin, F. and D. Paillard (2003), Amplitude and phase of glacial cycles from a conceptual model, Earth Planet. Sci. Lett., 214, 243-250.
Saltzman, B., and Verbitsky, M., 1994. Late Pleistocene climatic trajectory in the phase space of global ice, ocean state, and CO2: Observations and theory.  Paleoceanography, 9: 767–779.
Shackleton, N. J., A. Berger, and W. R. Peltier, 1990. An alternative astronomical calibration of the Lower Pleistocene timescale based on ODP site 677, Trans. R. Soc. Edinburgh, Earth Sci., 81: 251-261.

Americans and French begin new cold war in West Africa

Stuck in traffic this afternoon in Accra (the traffic is an entire book in itself). BBC suddenly stopped, and over the airwaves came Voice of America. I wouldn't normally listen to that but I wasn't doing much.

One of the top stories was a long diatribe on the problems in Côte d'Ivoire. First up--it seems that Ouattara has launched "yet more" attacks against his former allies. It seems that the ongoing difficulties there are ultimately going to cause Ghana to become the Queen of Cocoa. There followed a fairly lengthy explanation of why this would be. According to VOA, the age of the average cocoa tree in Cote d'Ivoire is now thirty years, which is past the age of peak production. Farmers in Côte d'Ivoire have not bothered to replant their trees because of the interminable civil problems. Why invest in new trees if your crops may be destroyed or your land confiscated in the fighting?

But what jumped out at me was the repeated mantras about Ghana. For an article which was supposedly about Côte d'Ivoire, they sure talked up Ghana. Ghana is peaceful. Ghana is stable. Ghana will attract the foreign investment that Côte d'Ivoire desperately needs simply to prevent its cocoa production from entering a terminal decline. Ghana has had peaceful elections since 1992. Ghana. Ghana. Ghana.

On the other hand, any time Cote d'Ivoire was mentioned, it was in the context of ongoing instability (now going on ten years), and implied instability of the new President, Ouattara. Infrastructure failing. Instability. Lack of investment. Cocoa trees dying. Production falling. Cote d'Ivoire soon to be of limited importance in the cocoa market.

A few weeks ago, I wrote this:

The Americans have promoted Ghana as the gateway to West Africa. A part of this is the development of a highway system through Ghana from the coast to the interior. The French have their own ideas--that Ivory Coast will be the gateway to the interior.

Considering that Voice of America is US propoganda, it is quite clear that the US intends to make Ghana the gateway to West Africa, and they have already launched their first assault against the French in West Africa.

Update--May 7

Not sure if this is connected, but just as I was leaving West Africa, the French propoganda channel stated that the French Foreign Minister had threatened to withdraw French troops from Afghanistan. Admittedly, the exact wording was "Withdrawal of French forces from the Afghan mission has not been ruled out"--but recall that a diplomat will never come out and tell you what's on his mind. Instead of "I hate your guts and I'm going to kill you", a diplomat would say, "We have a fundamental difference of opinion, but I believe there is an elegant solution to our current impasse."

Water crisis in Accra easing

At our compound, a new low point was reached over the weekend. No electricity, no water, no internet. Just sit in the dark and drink. (Well, all right, we ran the generator for awhile).

Late Friday night, without prior warning, our internet server decided we had used up all our bandwidth for the month. Naturally it was impossible to add credit until Monday.

We had been warned about water shortages. The local water companies have been advertising that there would be shortages, and they advised their customers to accumulate water. Unfortunately these warnings were only issued after the water had stopped flowing. They reported that their pumping equipment has been damaged by the recent extreme fluctuations in power supply. It is a believable story.

The water companies have been reduced to delivering water by truck. One rumour that has been circulating is that the price premium for a truck delivery is so compelling that the water companies have decided to deliver water by truck rather than by pipe. This argument seems unlikely, as it is impossible to fulfill all demand for water by truck. The margin for the company of such a delivery is greater than it would be for piped water, but by piping they serve far more customers.

Now it's possible that the costs of supplying water by conventional means had risen more rapidly (possibly including the theft and other losses that pipelines in Africa face) than the price they were permitted to charge, forcing them to seek higher-margin means of delivery.

The government has stepped in to prevent price gouging by placing restrictions on water supplies for truck delivery. Usually the trucks take water from the Weija reservoir--a common sight from the coastal highway. But the government has passed regulations forbidding water withdrawals from that spot. They presumably believe that restricting truck traffic will force the water companies to restore normal pipeline deliveries. But the water companies dug in their heels. Let there be no water, and we’ll see who cracks first.

The water situation, shall we say, reached the boiling point. The entire metropolitan area as far as Tema has been without water since early March. Over two million people were waiting for relief.

By Thursday, our overhead tank was empty and we were down near the bottom of the underground tanks. We used generator power to pump our last water into the overhead tank. We called for a truck delivery on Friday, and were told the truck would not arrive until Saturday. In the old days (say, last month), the call was answered immediately as the pickup spot on the Weija reservoir is less than 30 minutes from the compound. Now the trucks go far afield, in this case Winneba (about 90 minutes away), which adds to the price.

They used the traditional first trick in inflationary times--kept the price constant but shrank the load. Normally the water fills all our tanks with about 1000 litres to spare. On this delivery, the (supposedly) same volume of water was insufficient to fill our tanks.

Since writing this a few days ago, water service has been restored. Nobody seems to know why.

Book review: G. Friedman's The Next Hundred Years

I have been going through George Friedman's book "The Next Hundred Years". It is a painful process. I find the book to be flawed on numerous levels, mainly by the intellectual limitations implied by Friedman's axiomatic geopolitical views. Nevertheless, there are some interesting points made, some of which are perhaps more interesting than the author intended.

In the book Friedman uses a series of logical arguments to predict geopolitical developments over the next century. This is a common approach in the social sciences, which distinguishes them from the natural sciences. The social sciences argue that they do not have the luxury of conducting experiments in the lab, which forces them to study their problems using formal systems.

There are natural sciences in which experimentation is similarily impossible. Paleoclimatology, historical geology, evolutionary biology--all of these involve trying to draw conclusions about complex systems on the basis of limited observations. It is very difficult to draw conclusions, and impossible to make predictions; but whenever there have been problems in these sciences, they have arisen by a reliance on axioms (e.g., increased CO2 necessarily leads to warming).

A logical (or formal system) is characterized by its rules of inference, its axioms, and its definitions. All of these characteristics serve as irreducible statements, which must be assumed true. They cannot be proven to be true. If you reject the assumptions, you reject all the conclusions. Any flaws in the axioms will be carried through to the conclusions.

Friedman defines civilization as the state achieved by a culture making itself open to the ideas of other cultures. He further defines barbarism and decadence as intervals in which the culture closes itself to external ideas; the first before they have become civilized, the second, afterwards.

I have seen better definitions of civilization elsewhere--principally, civilization involves division of labour, leading to surpluses, a portion of which is used to improve one's own standard of living and a portion of which is used to build something for the future--whether it be temples, roads, bridges, factories.

I would argue that a civilized people save and invest in future-oriented development rather than simply consuming their wealth. (This idea comes from a delightful article which argued that the very pinnacle of civilized behaviour is the making of port, which appeared on Lewrockwell.com, but which I am currently unable to find). Under barbarism, surpluses are consumed. In this schema, decadence might be the state of barbarism in which a formerly civilized people consume not only all of their own surpluses, but also the capital accumulated and built by their more civilized forebearers.

Friedman argues that cultures start off as barbaric, become civilized, and then decadent. He believes that America is still in its barbaric phase, and will become civilized sometime in the next century. Although I find this a charming scenario, I have to disagree. By the definition I have used, America was civilized in the past two centuries, when it built its infrastructure and factories. Currently consumption dominates the economy; and as formerly paved roads are being torn up and replaced by gravel (as it is cheaper to maintain); as areas of major cities have services eliminated by cities which can no longer afford to provide them--America is clearly in the state of decadence.

America's decadence is driven by the excessive rewards given to financial engineers relative to civil engineers. If you were a bright fellow, there are far more rewards in the financial services industry (hedge fund manager etc.) than in manufacturing, mining, or other productive enterprises. But decadence need not be forever. Cultures can revitalize themselves by expanding their efforts to build for the future. Hopefully this will happen in America this century. In fact I think it likely will, once the current economic system completes its apparent collapse.

Back to Friedman. Two axioms really stand out in his early arguments.

1) Economic power flows from military power.

2) War is inevitable, so long as humans act in their own interests.

Observation suggests flaws in the first two assertions. First of all, it is a virtual certainty that it is military power which flows from economic power, rather than the reverse. Invading and plundering your more prosperous yet peaceful neighbours only works for so long. The plunder will not likely lead to lasting economic power, and you either run out of prosperous neighbours or else they arm themselves.

Great economic power makes it possible to become a great military power. If military power created economic power, than the Soviet Union would have had a much stronger economy. 

I would also argue that war does not arise inevitably from competing human interests. For instance Mr. Chu, who runs the corner store down the street, and I have differing interests, but we do not need to fight. As my time-money preferences vary, sometimes I will agree to pay Mr. Chu's prices, and other times I will walk a few more blocks to the grocery store. Most people recognize that there is more to be gained by mutually agreeable trade, which adds value, than through war, which consumes and destroys capital.

(You may wonder why I kept reading this book. Well, truth to tell, our compound here in Ghana has recently suffered a direct lightning strike, blowing up the TV, greatly limiting my entertainment choices. And bandwidth being what it is, at least books are available).

Given the difficulties I have with his axioms and definitions, it is unlikely I would agree with his conclusions. Nevertheless, some do appear to be viable.

Friedman expects Turkey to become a significant regional power. I agree.

He expects China to stumble and break up. I agree that there may be a stumble, particularly given their demographic problems, and agree that there is a chance that the economic disparity between urban and rural populations will strain the country, but think that the probability of China breaking up is less than Friedman projects. I see no reason why China's future stumbles should be worse than America's early economic stumbles.

Friedman expects Japan to become the dominant Asian power. I disagree, as their demographic situation is the worst in the world (except possibly for Russia). With their general lack of resources and impending demographic collapse, they are in the midst of significant decline.

He expects America to be the dominant global power throughout the next century. I don't agree because the economic and regional disparities make America about as likely to break up as China.

Friedman believes that the conflict between the US and the jihadists (sic.) is nearing its end, as America has nearly achieved its strategic objectives. At first glance, this statement seems to be completely at odds with reality. Despite talk of winding down its military operations in Iraq, the sheer size of the bases and US embassy there suggest that the military will continue to operate there in large numbers. As their simple presence in Iraq generates more enemies, they will never cease combat operations in Iraq until they completely withdraw from the country. Yet it is their presence in Iraq which is their strategic objective.

But this view presupposes that American foreign policy has the goal of establishing order in Iraq (or Afghanistan, or Libya, etc.). Friedman asserts that this is not America's goal. In what is the most useful bit of information gleaned from this book, he asserts that America's actual goal is to sow chaos and confusion throughout Eurasia in order to ensure that no other global power arises. While I find this a nearly perfect explanation for American foreign policy in the region, I question his assertion that it is "right" for a global power like America to condemn many millions of people to lives of poverty and conflict.

He states that Al-Qaeda has comprehensively failed in its strategic objectives, which he claims are to weaken US influence enough in the Middle East so that US-friendly regimes like Egypt, Tunisia, etc. would overthrow their governments. Never gonna happen in a hundred years, according to Friedman. Actually it only took two (once they allied themselves with Ben Bernanke).

As I recall, Bin Laden stated Al-Qaeda's objectives as threefold: 1) expulsion of US forces from Saudi Arabia (not necessarily US influence over the leadership); 2) resolution of the Palestinian situation; and 3) higher oil prices. They have achieved goal 1, and made progress on goal 3 (I believe his target was $300 per barrel--so I would not classify their actions as a strategic failure. In fact they have done remarkably well, given the imbalance in power between the two adversaries.

Friedman has a lot more to say in the later chapters about Russia; inevitable wars between America and major regional powers (which would not be necessary if the US didn't keep a finger in every pie) and a demographic/economic showdown with Mexico. I am afraid that I have to classify much of the book as wishful thinking rather than hard analysis.

A little more on rhomboidal rills

Spent a pleasant day on Axim beach watching the rhomboidal rills form while at sea Ivoiran refugees streamed eastward.

The beforms on this particular beach are not as impressive as the last time, due to the lack of black/purple heavy minerals. In these forms the sorting is mainly seen in variations in grain size.

No scale. My bad. The largest features are about 20 cm in length.

The more detailed view above shows one of the larger rhomboidal rills left of centre breaking up into many smaller features.

Bandwidth won't allow any of the films to be posted just now, but one thing that has become clear is that even after the wave has receded, there is a very slow flow of coarse light grains down the edges of the rills.

Self-organization in the flow showed up well in the photo below.

Self-organized branching flows during wave recession.

Ivoirans escaping by sea to Ghana

When I came out to Axim, western Ghana a few days ago, I expected to see many refugees from Cote d'Ivoire fleeing along the highway. But the road was completely dead--the border had been closed last week and nobody was getting through (although three weeks ago there was a steady stream of vehicles loaded with people and cocoa heading into Ghana).

The refugees are escaping by sea.

These fishing vessels have been cruising past the hotel every few minutes. The hotel staff tell us that this has been going on for weeks. The vessels are actually from fishing towns in Ghana, particularly Busua, Dixcove, Cape Coast, and points even farther to the east. The fishing in those areas has lately been poor, and the fisherman have found a new source of income.

They motor to Abidjan, or other coastal towns, pick up paying customers, and bring them back to their home port. The refugees are primarily Twi-speaking groups from the south of the country, who are culturally similar to the southern Ghanaians. They will also have been Gbagbo supporters.

I have to admit that it isn't as crowded as I would have thought it would be, but it is a long journey, and the boats are known to break in half. When fishing they are usually farther offshore.

Update April 12

According to today's Daily Graphic (a Ghanaian newspaper), the escaping refugees were not Ivoirans, but Ghanaians (more than a million of whom live in Cote d'Ivoire in the area surrounding Abidjan). They have been trapped there since the border was closed last week. There were harrowing descriptions of life for these Ghanaians (food cut off, people starving, dead children in the streets). As some of the fishing boats decided to return to Sekondi, many of these Ghanaians bought passage.

You can lead a horse to water . . .

A brief recap:

Damage to the economic system is causing leverage to fail. The prices of heavily leveraged assets (including house prices and the stock market are in danger of collapsing.

In order to stave off this collapse, central banks are using the usual techniques as well as some brand new ones to create more money in order to prop up these asset values and convince you and me that we are still well-off.

Unfortunately, large amounts of this money are being used to buy commodities, which is forcing their prices up. This makes you and me feel we are not so well-off, and this is bad from the central banks' perspective. The central bankers would like us to all buy bigger houses and invest in the stock market.

Notice that it is appearances that matters.

There has been much whining from central bankers about our complaints about food and energy prices (typical response--"shut up and buy and iPad2".)

All of this proves the old saying: You can lead a horse to water but you can't stop him from pissing in the pond."

A plague on all their houses--Ghanaian perspectives on the Ivoiran crisis

Recent news has the UN carrying out air strikes in Abidjan. But the idiots are bombing the forces that are defending the city from an invading force. Killings continue.

In this article I will present the prevailing understanding that lives on the street in Ghana (particularly western Ghana). I cannot verify the truth of these statements, and acknowledge the possibility that there are many misconceptions. However I believe their stories may add a different perspective than may be presented in western news media.

Many Ghanaians feel that both Ouattara and Gbagbo are unfit to preside over their country. They have both disqualified themselves by resorting to violence. Even in the case of Gbagbo (whom most Ghanaians believe is the wronged party in the dispute--see below), Ghanaians feel that once it became clear that his resistance would lead to bloodshed, he should have become a great statesman, yielded power, and waited for the next election.

Ouattara is from the north of Ivory Coast. He is Ivoiran, but of Burkinese descent, as are many of the Mosi-speaking peoples of Ivory Coast. In the last election, Ouattara benefitted tremendously from miraculous voting patterns, in which villages of 500 delivered thousands of votes (unsubstantiated statement, probably from Ghanaian news article). Allegations of voter fraud were not investigated. He has won the election, but that does not give him the right to massacre those who voted against him. (If it does, then I pray Harper doesn't win next month in Canada).

On his last visit to the African Union, the Nigerians offered to arm him. The Ghanaians were not in favour of him, so the arms were shipped by a northerly route, through Burkina Faso (unsubstantiated statements).

Gbagbo is from the south, representing peoples who generally originated in Ivory Coast. In a sense, he is following a tradition, in that when he first won an election, the former President also resisted leaving office, and both sides resorted to violence. Gbagbo reduced French involvement in Ivory Coast, leading to a resurgence of formerly suppressed Ivoirian culture. Under the French, the only culture in Ivory Coast was French.

The perception of Ghanaians is that Ouattara will return Ivory Coast to France. Hence the arrival of French troops to defend "French interests" including the airport.

The Americans have promoted Ghana as the gateway to West Africa. A part of this is the development of a highway system through Ghana from the coast to the interior. The French have their own ideas--that Ivory Coast will be the gateway to the interior.

Although cocoa has been much discussed in the media, there has been much less dicussion about the offshore oil, which has been the subject of exploration and exploitation for much longer than the recently discovered oil off Ghana.

The attack on Abidjan was remarkably well coordinated, with simultaneous strikes on key facilities, such as the Presidential palace, the radio and TV stations, and arms depots. Compare this to the mob-like efforts of the Libyan rebels. There was a three-day period in which Ouattara forces massed outside of Abidjan, as supplies and ammunition were brought to the front, and plans were made. Once again, this is a suspiciously well-disciplined mob.

A helpful summary

Ouattara--bad
Gbagbo--bad
The French--bad
The UN--bad

A plague on all their houses!

A note about stepping back for the good of the country. When elections were held in Ghana in November of 1996, I recall being amazed at the distribution of votes. All through the thinly populated northern regions there were enormous numbers of votes for Rawlings' party (the NDC). Furthermore, the votes in the north were unanimously in favour of the NDC (e.g., 65,912 to 2). Kuffour's party (the NPP) decided not to contest the results. I remember the tension leading up to that announcement and the tremendous relief afterwards. The NPP were rewarded by winning the next election four years later.

Massacres in West Africa: where are the airstrikes?

The situation in Cote d'Ivoire is deteriorating rapidly. A major massacre is reported at Duekoue, in the western part of the country. I don't know what news you are receiving, but what is being reported here (in Ghana) as the slaughter of Gbagbo supporters (who by all accounts lost the election) by Outtara supporters.

In Libya, Gaddhafi had only promised to massacre civilians. Here, actual massacres are already taking place.

Will there be any action on the part of the UN or other interested parties, or do they only protect civilians on the right side?

I am expecting to travel to Nzemaland, near the border between Ghana and Cote d'Ivoire early next week and will try to look in on the refugee situation.

In the heart of Agbogbloshie market

Aargh! The bandwidth! This post has taken five days to put together.

Power has been out in Kokrobitie for a couple of days. The official story is that they are working on the high-tension lines, so all power needs to be shut down. Rumours are that this will go on for seven weeks.

What you need for midnight blogging in the black-out. 

Laptop with internet stick, 12-V battery and power inverter, 

wind-up lantern, beer. The pizza arrived about ten minutes later.

Our photos today are from the heart of Agbogbloshie market, which as we have seen before is packed with more onions than the mind can comfortably conceive.

The place is loud. And the moment you arrive you are besieged by people determined to sell you onions.

What do you feel like? Dark red, high antioxidant onions? Sweeter white onions? Holland onions?


There really is a lot of food here. It just happens that a lot of it is onions.

It isn't all onions. Garlic in Ghana is really pungent. Not sure if it is the selenium.

I'll post more photos if bandwidth improves.